3Week 38

3.1 Readings for this week's lectures

Sections 7.3, 7.12 and 14.1 in the textbook.

3.2 Readings for this week's exercises

Sections 6.8 – 6.11 and 8.5 in the textbook.

3.3 Notes

Leibniz's notation

In Leibniz's notation the derivative $f'(x)$ of a function $y = f(x)$ is written in one of the following ways:

$f'(x) = \frac{\mathrm{d}f}{\mathrm{d}x} = \frac{\mathrm{d}y}{\mathrm{d}x}$

Rules for differentiation

In Leibniz's notation the rules for the derivatives of a sum/difference, a product and a quotient of two functions are expressed like this:

$\begin{aligned} {}& \frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} \left( f(x)\pm g(x) \right) = \frac{\mathrm{d}f}{\mathrm{d}x} \pm \frac{\mathrm{d}g}{\mathrm{d}x} \\[3ex] {}& \frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} \left( f(x)\cdot g(x) \right) = \frac{\mathrm{d}f}{\mathrm{d}x} \cdot g(x) + f(x)\cdot \frac{\mathrm{d}g}{\mathrm{d}x} \\[3ex] {}& \frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} \left( \frac{f(x)}{g(x)} \right) = \frac{ \frac{\mathrm{d}f}{\mathrm{d}x} \cdot g(x) - f(x)\cdot \frac{\mathrm{d}g}{\mathrm{d}x}} {\left( g(x) \right)^2} \end{aligned}$

In the prime notation the rules look as follows

$\begin{aligned} {}& F(x) = f(x)\pm g(x) \quad\Longrightarrow\quad F'(x) = f'(x)\pm g'(x)\\ {}& F(x) = f(x)\cdot g(x) \quad\Longrightarrow\quad F'(x) = f'(x)g(x) + f(x)g'(x)\\ {}&F(x) = \frac{f(x)}{g(x)} \quad\Longrightarrow\quad F'(x) = \frac{f'(x) g(x) - f(x) g'(x)}{\left( g(x) \right)^2} \end{aligned}$

Chain rule

For a composite function $F(x) = f(g(x))$ , and with $y=f(u)$ and $u=g(x),$ the chain rule can be written as:

$\begin{aligned} F'(x) &= f'(g(x)) \, g'(x) \\[3ex] \frac{\mathrm{d}y}{\mathrm{d}x} &= \frac{\mathrm{d}y}{\mathrm{d}u}\frac{\mathrm{d}u}{\mathrm{d}x} \end{aligned}$

Higher order derivatives

$\begin{aligned} f''(x) &= \frac{\mathrm{d}^2f}{\mathrm{d} x^2} \\ f^{(3)}(x) &= \frac{\mathrm{d}^3f}{\mathrm{d} x^3} \\ & \dots \\ f^{(n)}(x) &= \frac{\mathrm{d}^nf}{\mathrm{d} x^n} \end{aligned}$

3.4 Exercises

In this exercise we consider how capital grows after investing an amount that yields continuous interest.

Let $F(t) = Be^{rt}$ denote the total capital as a function of time $t$ measured in years since the initial investment. $B$ is the amount initially invested in million EUR and $r$ is the interest rate. If the interest is 15%, then $r=0.15$ .

It is fine to give your answer in terms of $e$ , so hypothetical answers could be, e.g., $5e$ or $\frac{1}{2}e^{0.2}$

If the initial capital invested is 2 million EUR and the interest rate is 10%, what is the total capital after 10 years?
What is the rate of increase of capital after 10 years?

Calculate the derivatives below:

$\displaystyle \frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} \frac{e^x - 1}{x^2}$
$\displaystyle \frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} \left( x^2 + \ln(x^2) \right)$
$\displaystyle \frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} \frac{\ln x}{x}$
$\displaystyle \frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} \frac{x}{\ln x}$
$\displaystyle \frac{\mathrm{d}\phantom{y}}{\mathrm{d}y} \left( e^{y} \cdot \ln y \right)$
$\displaystyle \frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} e^{-2x^3-4x}$

Find the derivative of the functions below, both with and without using the chain rule.

$f(x) = \ln(x^5)$
$g(x) = (x^2-2)^2$

In each case, which method is easier?

Calculate $\frac{\mathrm{d}y}{\mathrm{d}x}$ where $y = \sqrt{u}$ and $u = 3x^2 + \tfrac{1}{2}x$ .

Use the chain rule to calculate the derivatives below.

$\displaystyle\frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} (-x^3 + 7x + 5)^9$ .
$\displaystyle\frac{\mathrm{d}\phantom{t}}{\mathrm{d}t} 5(-3t^3 + 7t)^{-5}$ .
$\displaystyle\frac{\mathrm{d}\phantom{x}}{\mathrm{d}x} \frac{-3}{(x-3x^2)^3}$ .

A variable $z$ is a function of another variable $q$ , that, furthermore, is a function of the variable $t$ . The relations between the variables are given by the following expressions:

$z = p(q) \qquad q = w(t).$ Construct the correct expressions for the chain rule using both Leibniz's notation and the prime notation, by dragging the correct items onto the empty boxes below.

$\displaystyle\frac{\mathrm{d} z}{\mathrm{d} t} = \,$

$\,\cdot\,$

$z'(t) = \,$

$\Bigg($

$\Bigg)$ $\,\cdot\,$

$\Bigg($

$\Bigg)$

$p$

$z$

$t$

$\displaystyle\frac{\mathrm{d} z}{\mathrm{d} p}$

$\displaystyle\frac{\mathrm{d} p}{\mathrm{d} q}$

$\displaystyle\frac{\mathrm{d} q}{\mathrm{d} t}$

$w(t)$

$p'$

$w'$

Find $f'(x)$ , $f''(x)$ and $f^{(3)}(x)$ for the function $f(x) = 3x^3 - 5x^2 + 27.$

Calculate the following derivatives.

$f''(x)$ , where $f(x) = x^2 e^{-2x}$
$g''(y)$ , where $g(y) = (1-3y^3)^7$
$\displaystyle\frac{\mathrm{d}^2y}{\mathrm{d}x^2}$ , where $y = \sqrt[6]{x}$

Let $f(t)$ denote the turnover of Maersk Line in the year $t$ .

Connect the statements below with the correct mathematical expressions by dragging the expressions onto the right boxes.

a) The turnover is falling, but the rate of the fall is decreasing

b) The turnover is growing, and it grows more and more with time

c) The turnover is growing, but it grows less and less with time

d) The turnover is falling, and the rate of the fall increases

$f'(t)>0$ and $f''(t)>0$

$f(t)=0$ and $f'(t)<0$

$\frac{df}{dt}<0$ and $\frac{d^2f}{dt^2}>0$

$f'(t)<0$ and $f''(t)<0$

$f'(t)>0$ and $f''(t)<0$

A function $u$ is given by: $u(v) = \sqrt{v^2 + 1}$ .

What is $\displaystyle\frac{\mathrm{d} u}{\mathrm{d} v}$ ?

The result can be written in several ways. Try to express your result in terms of $u$ and $v$ and simplify as much as possible.

$\frac{v}{u}$

$uv$

$\frac{1}{2u}$

$\frac{\sqrt{v}}{2u}$

What is $\displaystyle\frac{\mathrm{d}^2 u}{\mathrm{d} v^2}$ ?

The result can be written in several ways. To find the correct answer below, try to rewrite your expression using the result above, after taking the derivative, and use the relation $u = \sqrt{v^2 + 1}$ to express $u^2$ in terms of $v$ after simplifying.

$\frac{uv - vu'}{u^2}$

$\frac{u - vu}{u^2}$

$\frac{1}{u^3}$

$\frac{{u^2-v^2}}{u^2}$

Below are shown graphs of functions with different combinations of signs of 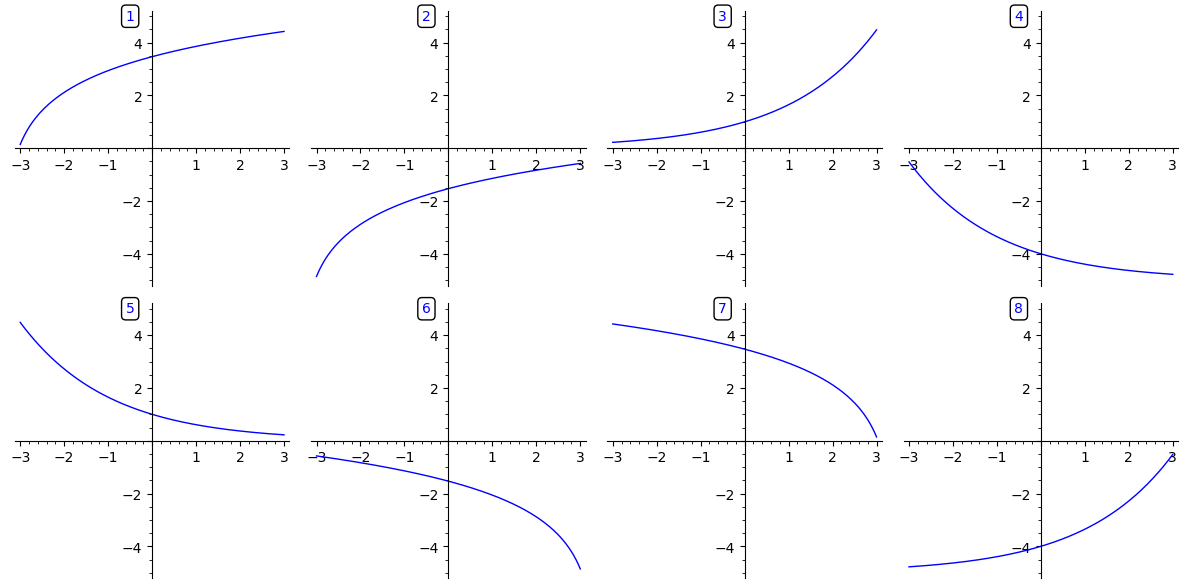$f(x)$ , $f'(x)$ and $f''(x)$ .

Drag the numbers corresponding to the graphs onto the empty boxes, so that they match the signs of $f(x)$ , $f'(x)$ and $f''(x)$ .

$f(x) > 0$ , $f'(x) > 0$ , $f''(x) > 0$

$f(x) > 0$ , $f'(x) > 0$ , $f''(x) < 0$

$f(x) > 0$ , $f'(x) < 0$ , $f''(x) > 0$

$f(x) > 0$ , $f'(x) < 0$ , $f''(x) < 0$

$f(x) < 0$ , $f'(x) > 0$ , $f''(x) > 0$

$f(x) < 0$ , $f'(x) > 0$ , $f''(x) < 0$

$f(x) < 0$ , $f'(x) < 0$ , $f''(x) > 0$

$f(x) < 0$ , $f'(x) < 0$ , $f''(x) < 0$