11Week 46

11.1 Readings for this week's lectures

Sections 10.5-10.6 in the textbook.

11.2 Readings for this week's exercises

Sections 10.1-10.4 in the textbook.

11.3 Notes

Definitions

The indefinite integral:

$\int f(x)\, {\mathrm{d}}x = F(x) + C$

The definite integral:

$\int_a^b f(x)\, {\mathrm{d}}x = \bigg|_a^b F(x) = F(b) - F(a)$

General rules

$\displaystyle\int af(x) \, {\mathrm{d}}x = a\displaystyle\int f(x)\, {\mathrm{d}}x$

$\displaystyle\int a \, {\mathrm{d}}x = ax + C$

$\displaystyle\int x^a \, {\mathrm{d}}x = \frac{x^{a+1}}{a+1} + C, \quad a\neq -1$

$\displaystyle\int \frac{1}{x}\, {\mathrm{d}}x = \ln|x| + C,\quad x\neq 0$

$\displaystyle\int e^x\, {\mathrm{d}}x = e^x + C$

$\displaystyle\int e^{ax}\, {\mathrm{d}}x = \frac{1}{a}e^{ax} + C, \quad a\neq 0$

$\displaystyle\int a^{x}\, {\mathrm{d}}x = \frac{1}{\ln a}a^x + C, \quad a>0,\, a\neq 1$

$\displaystyle\int \ln x\, {\mathrm{d}}x = x\ln x - x + C, \quad x>0$

Rules for definite integrals

$\displaystyle\int_a^b f(x)\, {\mathrm{d}}x = -\int_b^a f(x)\, {\mathrm{d}}x$

$\displaystyle\int_a^a f(x)\, {\mathrm{d}}x = 0$

$\displaystyle\int_a^b \alpha f(x)\, {\mathrm{d}}x = \alpha \int_a^b f(x)\, {\mathrm{d}}x$

$\displaystyle\int_a^b f(x)\, {\mathrm{d}}x = \int_a^c f(x)\, {\mathrm{d}}x + \int_c^b f(x)\, {\mathrm{d}}x$

$\displaystyle\int_a^b f(x) + g(x)\, {\mathrm{d}}x = \int_a^b f(x)\, {\mathrm{d}}x + \int_a^b g(x)\, {\mathrm{d}}x$

11.4 Problems

Find the following indefinite integral:

$\int (14x^2-8x+2)\, {\mathrm{d}}x$

Find the following indefinite integral:

$\int \frac{1}{2x^4}\, {\mathrm{d}}x$

Find the following indefinite integral:

$\int (x^4 + 5e^{-2x} - \sqrt{x})\, {\mathrm{d}}x$

Find the following indefinite integral:

$\int \frac{u^6-4u^3+12}{u^4}\, {\mathrm{d}}u$ Then, find the following definite integral:

$\int_{1}^{2} \frac{u^6-4u^3+12}{u^4} \, {\mathrm{d}}u$

You are attending a UN climate summit and have been asked to match mathematical expressions to sentences describing the same thing.

Let $f(x)$ denote the Earth's total $\mathrm{CO}_2$ emissions i the year $x$ measured in millions of tonnes.

If the Earth's total emissions of $\mathrm{CO}_2$ in the year 2022 is 10,000 million tonnes, this means that $f(2022) = 10^4$ .

Connect the expressions below with the corresponding mathematical expressions by dragging them into the empty boxes.

a) $\mathrm{CO}_2$ emissions were higher in 2000 than in 2010:

b) $\mathrm{CO}_2$ emissions peaked in 2005:

c) The latest thirty years up to and including 2022 have resulted in emission of the same amount of $\mathrm{CO}_2$ as in all of the previous period since the start of the industrialisation in 1850:

d) In 2012 emissions were approximately 240 million tonnes less than the year before:

$f(2012) < 240$

$\displaystyle\int_{1850}^{1992} f(x) \, \mathrm{d}x = \int_{1992}^{2022} f(x) \, \mathrm{d}x$

$f'(2000) > f'(2010)$

$f(2005) = 0$ og $f''(x) > 0$

$\displaystyle\int_{1850}^{1992} f(x) \, \mathrm{d}x = \int_{0}^{30} f(x) \, \mathrm{d}x$

$f'(2005) = 0$ og $f''(x) < 0$

$f'(2012) = -240$

$f(2010) < f(2000)$

$f(0) = 2005$

$f'(2005) = 0$ og $f''(x) > 0$

Find the following definite integral:

$\int_{0}^{3}(2x^2+4)\, {\mathrm{d}}x$

A definite integral can be calculated as the limit of a sum, where the number of terms in the sum approaches infinity. This definition of a definite integral is called the Riemann integral. The integral can be written as (see p. 371 in the textbook):

$\int_a^b f(x) \,\mathrm{d} x = \lim_{n\to\infty} \sum_{i = 0}^{n - 1} f(\xi_i) \Delta x_i,$ where $\xi_i$ is a value in the $i$ 'th interval and $\Delta x_i$ is the width of the interval.

Below is shown an example where $f(x) = 2x^2+4$ . In the example the limit $n\to\infty$ has not been taken, and the sum is calculated in the plot.

Explain the parameters controlled by the three sliders and relate them to the formula above.
Explain what happens to the sum when the number of terms becomes larger.
Determine the value of the integral in exercise (11.6) as well as you can by choosing appropriate values with the three sliders.
Did you get the same result as in exercise (11.6)?

Find the following definite integral:

$\int_{a}^{b}5e^{4t}\, {\mathrm{d}}t$

According to the Danish Meteorological Institute torrential rain is defined by 15 mm or more of rain in 30 minutes, while heavy rain, is when the amount of precipitation exceeds 24 mm in 6 hours over a larger geographical area.

Formulate the definitions of torrential rain and heavy rain mathematically. Introduce any variables functions, etc. that you need in order to express the definitions mathematically.

Find the following definite integral:

$\int_{0}^{4}\sqrt{3x}\, {\mathrm{d}}x$

Find the following definite integral:

$\int_{1}^{2}\frac{1+x^3}{x^2}\, {\mathrm{d}}x$

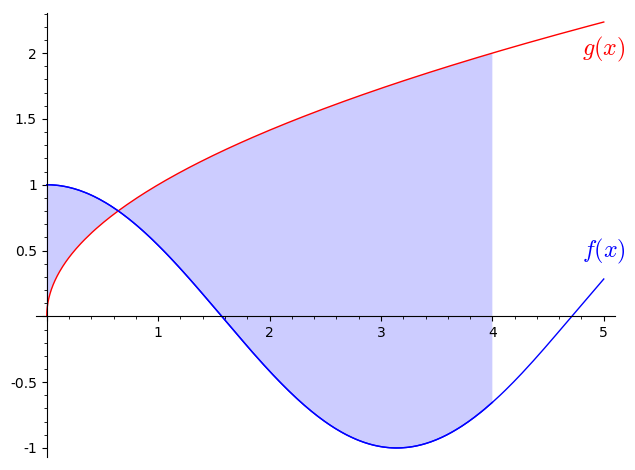
Drag the boxes below to the empty slots to construct an integral that is equal to the area coloured in the plot.

$\int_a^b h(x)\, \mathrm{d}x$

where $a =$

, $b =$

and $h(x) =$

$f(x)-g(x)$

$g(x)-f(x)$

$0$

$5$

$\vert g(x) - f(x) \vert$

$f(x) + g(x)$

$\sqrt{( f(x) - g(x) )^2}$

There is more than one correct solution. How many can you find?

Compute the area bounded by the graph of $f(x)= 1/x^3$ , the $x$ -axis and the two lines $x=-2$ and $x=-1$ .

$f(x) < 0$ for $x$ in $[-2,-1]$ .

Find the following definite integral:

$\int_{-3}^{3}(x-1)^2\, {\mathrm{d}}x$

Find the following definite integral:

$\int_{-2}^{2}(e^x-e^{-x})\, {\mathrm{d}}x$

(Previous exam problem)

Find the integral:

$\int_{5}^{10} e^{(x-5)}+\frac{1}{5}\, {\mathrm{d}}x$